• Github Repo
• Tools: OCaml (functional programming), OCaml Graphics

With a partner, I developed an OCaml program that solves maze and tile puzzles, displaying the process in OCaml graphics along with real-time performance metrics. Our software rested heavily on modular coding principles that allow for the reusability of functions across different user-inputted puzzles and various solving algorithms. The underlying implementations of the BFS, fast BFS, and DFS solvers are developed with stack and queue structures, and I used OCaml’s higher-order functions to write an abstract, efficient solver function that searches neighbors and stores their states.

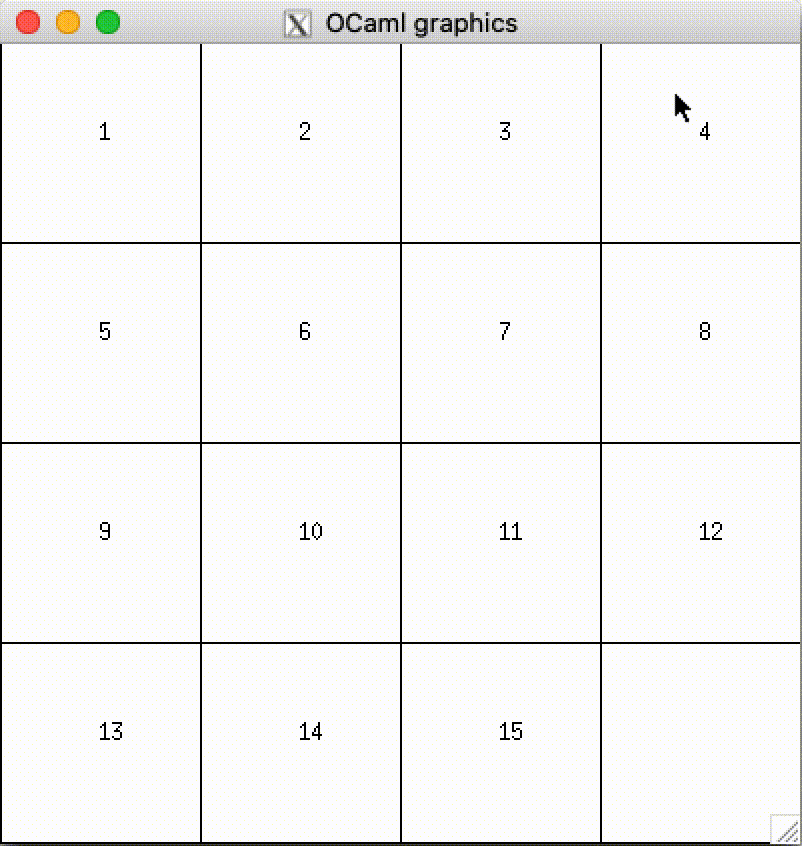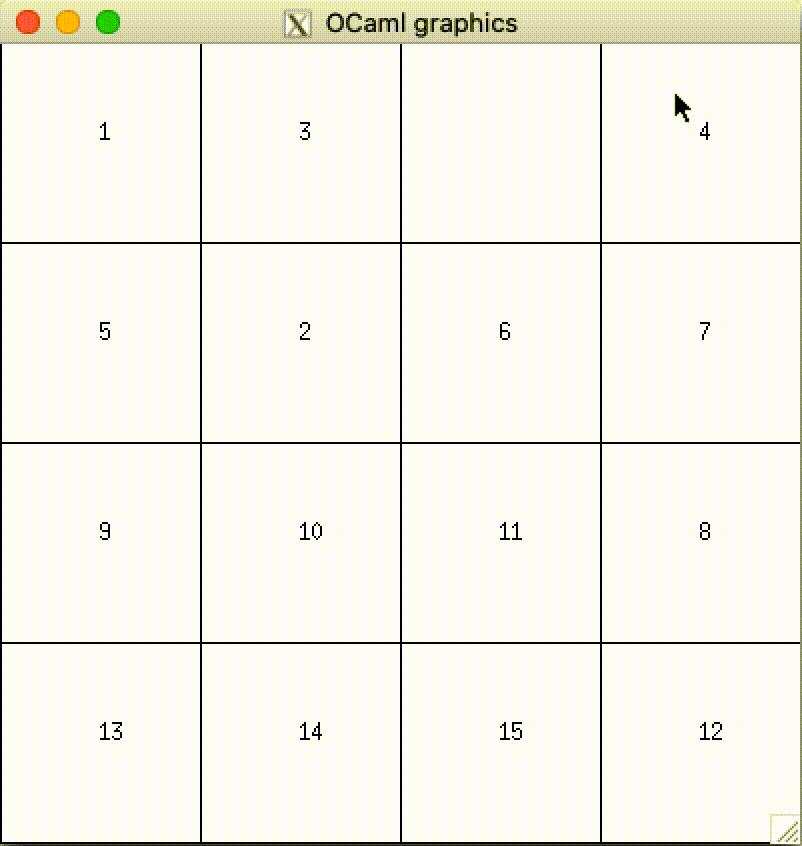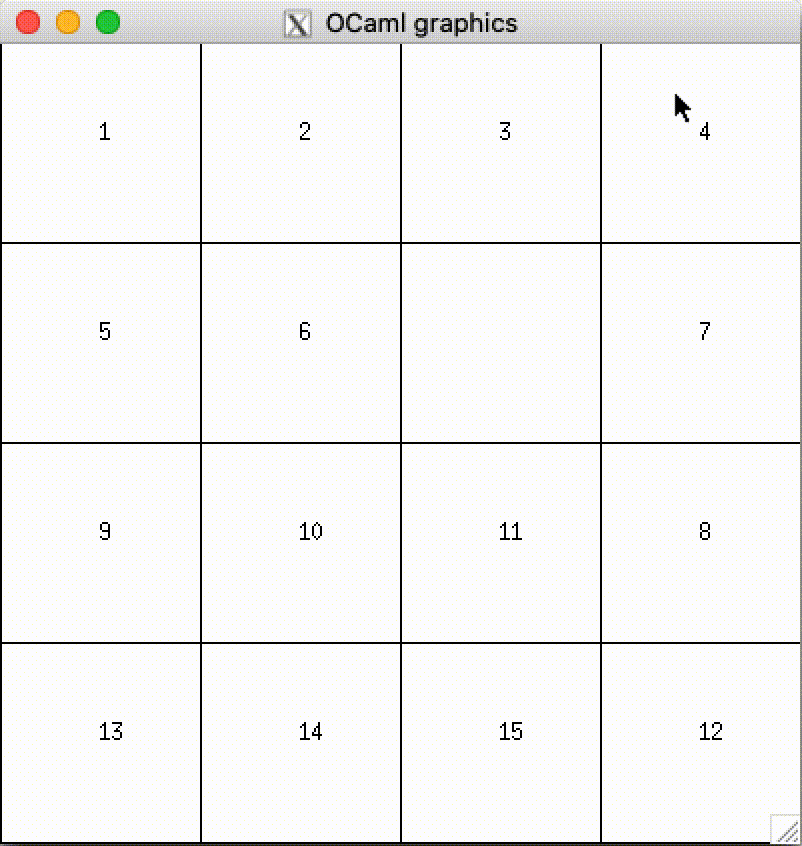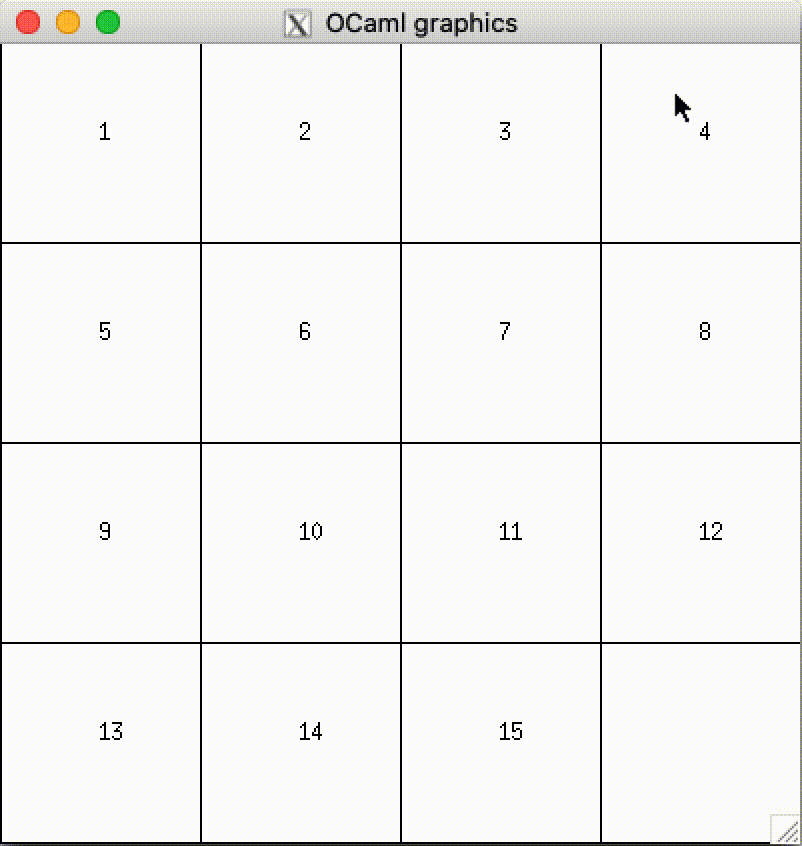
This program allowed us to run experiments and conduct research on the effectiveness of various solvers for different situations, across different sizes, directions, and solvability. I developed an extensive performance testing system that feeds the program a variety of puzzle types while outputting their performance. See below for a detailed analysis of the time complexity and comparisons across different solvers and kinds of puzzles, considering the tradeoffs for each.

Writeup of Experimental Results